A Piston Problem Twist
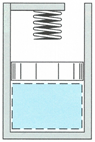
Consider the cylinder system shown in the figure below, which contains 1.5 g of air initially at 20°C and 120 kPa. At constant pressure, heat is then added to the air, raising the piston. The piston touches the spring when the air occupies a 2.5 liter volume. Heat in continually added until the air reaches a volume of 3.5 liters and a 2000K temperature. The universal gas constant is R(u) = 8.314 kJ/(kmol∙K) and the molar mass of air is 29 kg/kmol.
- How much work is done by the air?
- Sketch the process on a Pressure vs Volume diagram, labeling all the important states.
Expand Hint
$$$V=\frac{mRT}{P}$$$
where
$$V$$
is volume,
$$m$$
is mass,
$$R$$
is the universal gas constant,
$$T$$
is temperature, and
$$P$$
is pressure.
Hint 2
$$$W=P(V_2-V_1)$$$
$$$W=\frac{1}{2}(P_1+P_2)(V_2-V_1)$$$
where
$$W$$
is work,
$$P$$
is pressure, and
$$V$$
is volume.
$$$V_1=\frac{mRT_1}{P_1}=\frac{(1.5\cdot 10^{-3})(\frac{8.314}{29})(293)}{120}=1.05\:L$$$
where
$$V$$
is volume,
$$m$$
is mass,
$$R$$
is the gas constant,
$$T$$
is temperature, and
$$P$$
is pressure.
$$$P_3=\frac{mRT_3}{V_3}=\frac{(1.5\cdot 10^{-3})(\frac{8.314}{29})(2000)}{3.5\cdot 10^{-3}}=246\: kPa$$$
Solving for work,
$$$W_{1-2}=P_1(V_2-V_1)=120(2.5-1.05)=174\: J$$$
$$$W_{2-3}=\frac{1}{2}(P_2+P_3)(V_3-V_2)=\frac{1}{2}(120+246)(3.5-2.5)=183\: J$$$
$$$W=W_{1-2}+W_{2-3}=174+183=357\:J$$$
Pressure vs Volume diagram,
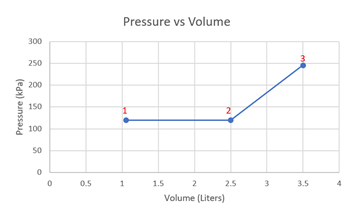
- 357 J
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Ideal Gas, Closed Thermodynamic System
Similar Problems from FE Section: PVT Behavior, First Law of Thermodynamics