Friction on a Ramp
Consider a current equilibrium state where a box is on an inclined ramp. The coefficient of static friction between the two is 0.5. As the ramp is pivoted upwards, what angle (degrees) will the box begin to slide?
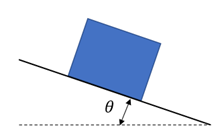
Expand Hint
Free Body Diagram:
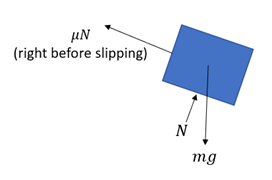
Hint 2
Analyzing the forces normal to the ramp:
$$$\sum F_{normal}=0=N-(mass\times gravity)cos\theta \rightarrow N=(mg)cos\theta $$$
First, draw the free body diagram on the box.
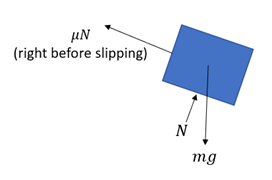
Analyzing the forces normal to the ramp:
$$$\sum F_{normal}=0=N-(mass\times gravity)cos\theta \rightarrow N=(mg)cos\theta $$$
Analyzing the forces tangent to the ramp:
$$$\sum F_{tangent}=0=\mu N-(mass\times gravity)sin\theta\to N=\frac{(mg)sin\theta}{\mu}$$$
Combining the two equations:
$$$\mu (mg)cos\theta =(mg)sin\theta$$$
$$$\mu=\frac{sin\theta }{cos\theta }=tan\theta =0.5$$$
$$$\theta =tan^{-1}(0.5)=26.6^{\circ}$$$
26.6°
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Friction
222. Sliding Down a Ramp
489. Ramp Sliding
Similar Problems from FE Section: Friction
222. Sliding Down a Ramp
489. Ramp Sliding
Similar Problems from FE Section: Impulse and Momentum
222. Sliding Down a Ramp
489. Ramp Sliding