Beam Distributed Load
In the below diagram, a distributed load of 10 N/m is applied to a section of Beam A-B. What is the reaction force a point B? Ignore the beam weight.
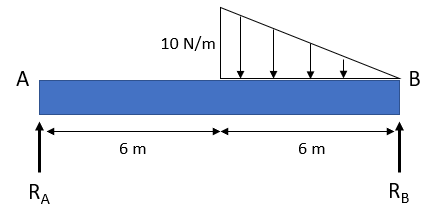
Expand Hint
The distributed force can be represented as a singular, concentrated force (F) acting through the triangle's centroid.
$$$F=\frac{1}{2}(base)(height)$$$
Hint 2
Calculate the sum of moments about Point A to determine the reaction at Point B:
$$$\sum M_{A}=0$$$
The distributed force can be represented as a singular, concentrated force (F) acting through the triangle's centroid. In addition, the triangle's area is the distribution's magnitude.
$$$F=\frac{1}{2}(base)(height)=\frac{1}{2}(6m)(10\frac{N}{m})=30\:N$$$
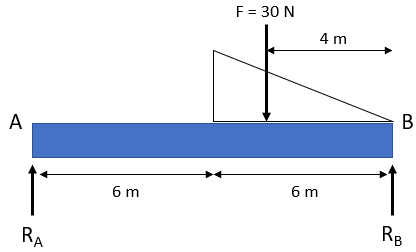
Next, let's calculate the sum of moments about Point A. We will be able to determine the reaction at Point B:
$$$\sum M_{A}=0=(force)(distance)$$$
$$$R_A(0m)+(12m)R_B-(30N)(8m)=0$$$
$$$(12m)R_B=(30N)(8m)$$$
$$$R_B=\frac{240N\cdot m}{12m}=20\:N$$$
20 N
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked