Garden Hose
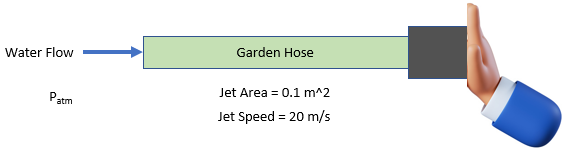
Consider a typical garden hose produces a horizontal jet of water (density = 1,000 kg/m^3). Estimate the minimum force required to stop the water flow with your hand by pressing it perpendicularly against the nozzle's opening.
Expand Hint
$$$Q=vA$$$
where
$$Q$$
is the flow rate,
$$v$$
is the velocity, and
$$A$$
is the cross sectional area.
Hint 2
Since the water jet is deflected perpendicularly, the required force must deflect the total horizontal momentum of the water.
$$$F=\rho Qv$$$
where
$$\rho$$
is the density,
$$Q$$
is the flow rate, and
$$v$$
is the fluid velocity.
Volumetric flow rate:
$$$Q=vA$$$
where
$$Q$$
is the flow rate,
$$v$$
is the velocity, and
$$A$$
is the cross sectional area.
$$$Q=vA=(0.1m^2)(20m/s)=2\:m^3/s$$$
Since the water jet is deflected perpendicularly, the required force must deflect the total horizontal momentum of the water.
$$$F=\rho Qv$$$
where
$$\rho$$
is the density,
$$Q$$
is the flow rate, and
$$v$$
is the fluid velocity. Thus,
$$$F=1,000\frac{kg}{m^3}\times 2\frac{m^3}{s} \times 20\frac{m}{s}=40,000\frac{kg\cdot m}{s^2}=40\:kN$$$
40 kN
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Impulse-Momentum Principle