Fluid Flow
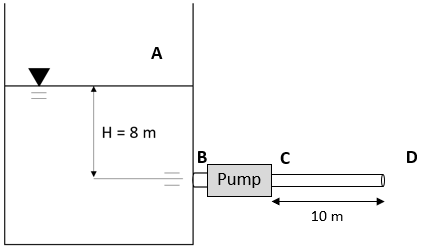
In the figure below, water is pumped through a 50 mm diameter pipe from a 8 m tall water tank that is opened to the atmosphere (Point A). The water leaves the pump at 3 m/s, and eventually exits the pipe into the atmosphere again at Point D. If the gauge pressure at Point B is 130 kPa, and 200 kPa at Point C, what is the pumping rate in m^3/min? Note the density of water is 1,000 kg/m^3.
Expand Hint
Flow rate:
$$$Q=Av$$$
where
$$A$$
is the cross sectional area of flow, and
$$v$$
is the average flow velocity.
Hint 2
The cross sectional area of the pipe:
$$$A=\frac{\pi }{4}D^2$$$
where
$$D$$
is the pipe’s diameter.
The cross sectional area of the pipe:
$$$A=\frac{\pi }{4}D^2=\frac{\pi }{4}(0.05m)^2=0.00196m^2$$$
Flow rate:
$$$Q=Av$$$
where
$$A$$
is the cross sectional area of flow, and
$$v$$
is the average flow velocity.
$$$Q=(0.00196m^2)(3\frac{m}{s})(\frac{60s}{min})=0.35\:m^3/min$$$
$$$0.35\:m^3/min$$$
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: The Continuity Equation
178. Fluid Velocity
366. Pump Efficiency
554. Fluid Density
Similar Problems from FE Section: Principles of One-Dimensional Fluid Flow
178. Fluid Velocity
348. Centrifugal Pump
366. Pump Efficiency
554. Fluid Density