Heat Pump Cycle
The shown figure describes a heat pump cycle using R-12a and its associated enthalpies. What is the coefficient of performance (COP)?
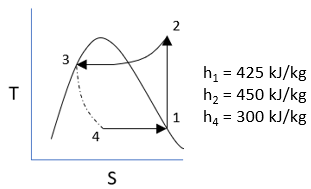
Expand Hint
For a heat pump cycle, the coefficient of performance is:
$$$COP_{hp}=\frac{h_2-h_3}{h_2-h_1}$$$
where
$$h$$
is the enthalpy.
Hint 2
For heat pumps and refrigeration cycles,
$$h_4=h_3$$
.
For a heat pump cycle, the coefficient of performance is:
$$$COP_{hp}=\frac{h_2-h_3}{h_2-h_1}$$$
where
$$h$$
is the enthalpy.
For heat pumps and refrigeration cycles,
$$h_4=h_3$$
. Thus,
$$$COP_{hp}=\frac{(450-300)}{(450-425)}\cdot \frac{kJ/kg}{kJ/kg}=\frac{150}{250}=6$$$
6
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Coefficient of Performance
249. Refrigeration Cycle
586. Fridge Cycle
Similar Problems from FE Sub Section: Refrigeration
249. Refrigeration Cycle
586. Fridge Cycle
Similar Problems from FE Section: Basic Cycles
249. Refrigeration Cycle
298. Carnot Cycle
454. Heat Pump
457. Heat Pump COP
586. Fridge Cycle
602. Carnot Fridge
Similar Problems from FE Section: Common Thermodynamic Cycles
249. Refrigeration Cycle
586. Fridge Cycle