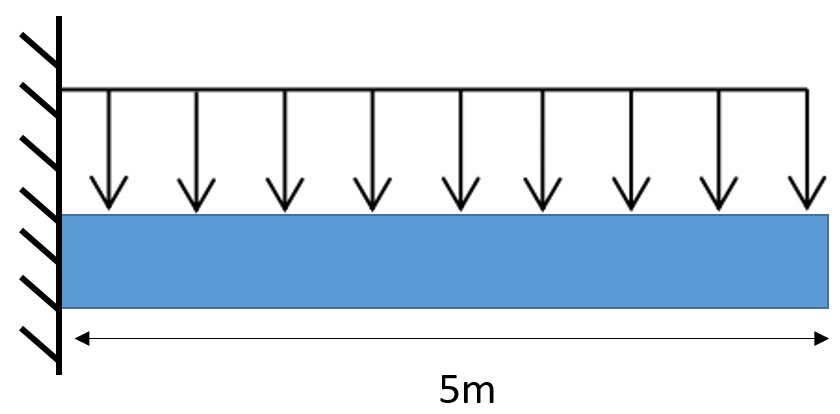
Cantilevered Beam
Calculate the deflection at the cantilevered beam's tip if a 7,000 N/m distributed load is applied. Assume E*I = 6.4x10^6 N*m^2.
Expand Hint
$$$\upsilon_{max}=\frac{-wL^{4}}{8EI}$$$
where
$$w$$
is the distributed load,
$$L$$
is the beam’s length,
$$E$$
is the modulus of elasticity, and
$$I$$
is the moment of inertia.
Hint 2
The final answer should be negative to indicate a downward deflection.
Referencing the cantilevered beam slopes and deflections section in the FE handbook (or any other ref table), the deflection formula is:
$$$\upsilon_{max}=\frac{-wL^{4}}{8EI}$$$
where
$$w$$
is the distributed load,
$$L$$
is the beam’s length,
$$E$$
is the modulus of elasticity, and
$$I$$
is the moment of inertia.
$$$\upsilon_{max}=\frac{-7,000N/m\cdot (5m)^{4}}{8\cdot 6.4\times 10^6N\cdot m^2}$$$
$$$\upsilon_{max}=-\frac{4,375,000}{51,200,000}\:m=-0.085\:m$$$
(Note: the negative sign (-) indicates the deflection is downwards.)
-0.085 m
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Cantilevered Beam Slopes and Deflections
267. Vertically Cantilevered
367. Superposition
Similar Problems from FE Section: Material Properties
267. Vertically Cantilevered
367. Superposition