Parallel Axis
In the below figure, what is the area moment of inertia about the X'-X' axis, which is 1 mm higher from the centroid?
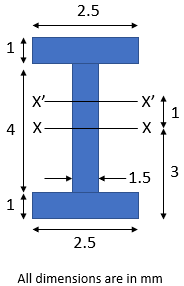
Expand Hint
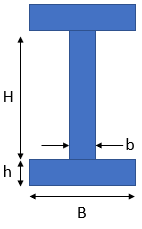
The area moment of inertia of an I-Beam along the horizontal axis passing through the body's centroid:
$$$I_{xx}=\frac{H^3b}{12}+2\left ( \frac{h^3B}{12}+\frac{hB(H+h)^2}{4} \right )$$$
where
$$H$$
is the flange-to-flange inner face height,
$$B$$
is the flange's width,
$$h$$
is the flange's thickness, and
$$b$$
is the web thickness.
Hint 2
Parallel Axis Theorem:
$$$I_x=I_{x_{c}}+d_{y}^{2}A$$$
where
$$d_y$$
is the distance between the new axis and the object’s centroid,
$$I_{x_{c}}$$
is the moment of inertia about the centroid axis,
$$A$$
is the total cross sectional area, and
$$I_x$$
is the moment of inertia about the new axis
The area moment of inertia of an I-Beam along the horizontal axis passing through the body's centroid:
$$$I_{xx}=\frac{H^3b}{12}+2\left ( \frac{h^3B}{12}+\frac{hB(H+h)^2}{4} \right )$$$
where
$$H$$
is the flange-to-flange inner face height,
$$B$$
is the flange's width,
$$h$$
is the flange's thickness, and
$$b$$
is the web thickness.
$$$I_{xx}=\frac{4^3(1.5)}{12}+2\left ( \frac{1^3(2.5)}{12}+\frac{1(2.5)(4+1)^2}{4} \right )$$$
$$$=8+2\left ( 0.2083+15.625 \right )=39.67\approx 40\:mm^4$$$
Because we want to find the moment of inertia about the parallel axis (X'-X') that is offset from the centroid (X-X) by 1 mm, we must use the Parallel Axis Theorem:
$$$I_x=I_{x_{c}}+d_{y}^{2}A$$$
where
$$d_y$$
is the distance between the new axis and the object’s centroid,
$$I_{x_{c}}$$
is the moment of inertia about the centroid axis,
$$A$$
is the total cross sectional area, and
$$I_x$$
is the moment of inertia about the new axis.
$$$I_{x'x'}=40mm^4+(1mm)^{2}[(2.5)(1)+(4)(1.5)+(2.5)(1)]mm^2$$$
$$$I_{x'x'}=40mm^4+11mm^4=51\:mm^4$$$
$$$51\:mm^4$$$
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Moment of Inertia Parallel Axis Theorem
196. Moment of Inertia
359. Axis Theorem
Similar Problems from FE Section: Moment of Inertia
196. Moment of Inertia
359. Axis Theorem