Rankine Cycle
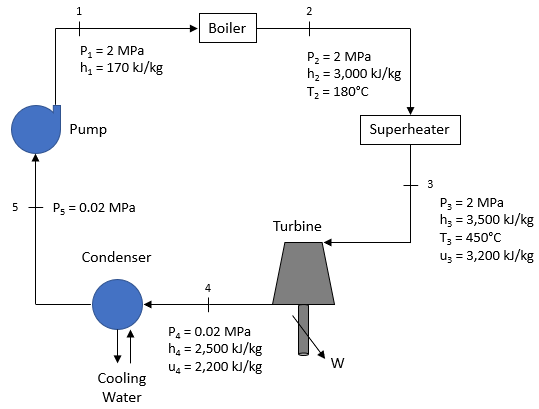
The Rankine cycle below is used to power a plant complex with water as the working fluid. If the pump’s isentropic efficiency is 75% and the fluid’s mass flow rate is 25 kg/s, how much power (kW) is required to drive the pump? Assume steady state and steady flow conditions. Also, disregard pressure losses and kinetic/potential energy effects. Note the density of water is 1,000 kg/m^3.
Expand Hint
The isentropic efficiency for a pump is
$$$\eta_{pump}=\frac{w_s}{w_{actual}}$$$
where
$$w_s$$
is the isentropic pump work per unit mass and
$$w_{actual}$$
is the actual pump work per unit mass.
Hint 2
The isentropic pump work for a constant density fluid is
$$$w_{s}=v(\Delta P)$$$
where
$$v$$
is the specific volume of the working fluid and
$$\Delta P$$
is the change in pressure.
The isentropic pump work for a constant density fluid is
$$$w_{s}=v(P_1-P_5)$$$
where
$$v$$
is the specific volume of the working fluid and
$$\Delta P$$
is the change in pressure. Since
$$v=\rho^{-1}$$
where
$$\rho$$
is the density:
$$$w_{s}=\frac{1(m^3)}{1,000kg}(2,000kPa-20kPa)=1.98\:kJ/kg$$$
The isentropic efficiency for a pump is
$$$\eta_{pump}=\frac{w_s}{w_{actual}}$$$
where
$$w_s$$
is the isentropic pump work per unit mass and
$$w_{actual}$$
is the actual pump work per unit mass.
$$$w_{actual}=\frac{w_s}{\eta_{pump}}=\frac{1.98kJ/kg}{0.75}=2.64\:kJ/kg$$$
Thus, to get the actual power required by the pump:
$$$\dot{W}_{actual}=w_{actual}\times\dot{m}=2.64\frac{kJ}{kg}\times 25\frac{kg}{s}=66\:kW$$$
66 kW
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Steady-Flow Systems
225. Boiler Pump
254. Turbine Power