Belt Friction
In the figure shown, a pulley is driven by a belt. What minimum coefficient of friction will prevent slipping between pulley and belt? Ignore centrifugal effects.
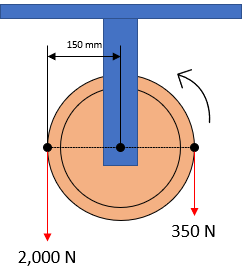
Expand Hint
Belt Friction:
$$$F_1=F_2e^{\mu \theta}$$$
where
$$F_1$$
is the applied force in the direction of impending motion,
$$F_2$$
is the applied force resisting impending motion,
$$\mu$$
is the static coefficient of friction, and
$$\theta$$
is the total angle of contact between surfaces in radians.
Hint 2
Unit Circle:
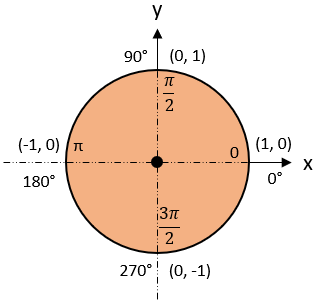
Belt Friction:
$$$F_1=F_2e^{\mu \theta}$$$
where
$$F_1$$
is the applied force in the direction of impending motion,
$$F_2$$
is the applied force resisting impending motion,
$$\mu$$
is the static coefficient of friction, and
$$\theta$$
is the total angle of contact between surfaces in radians. Recall a unit circle to determine
$$\theta$$
:
Therefore,
$$$2,000N=(350N)e^{\mu \pi}$$$
Solving for the coefficient of friction:
$$$ln(\frac{2,000}{350})=ln(e^{\mu \pi})=\mu \pi $$$
$$$\mu=\frac{1}{\pi}\cdot ln(\frac{2,000}{350})=\frac{1}{\pi} \cdot 1.743=0.56$$$
0.56
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Section: Belt Friction
385. Pulley + Belt
406. Pulley & Belt
579. Belt Force
584. Belt