Ln Base
Solve the shown logarithm:
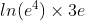
Expand Hint
The logarithm of
$$x$$
to the Base
$$b$$
is defined by
$$$log_b(x)=c$$$
where
$$b^c=x$$
.
Hint 2
Special definitions when
$$b=e$$
or
$$b=10$$
are:
- ln $$x$$ → Base = $$e$$
- log $$x$$ → Base = 10
The logarithm of
$$x$$
to the Base
$$b$$
is defined by
$$$log_b(x)=c$$$
where
$$b^c=x$$
. Special definitions when
$$b=e$$
or
$$b=10$$
are:
- ln $$x$$ → Base = $$e$$
- log $$x$$ → Base = 10
Since the natural log has a default base of
$$e$$
:
$$$ln(e^4) \times 3e=ln_e(e^4) \times 3e=(4)\cdot 3e=12e$$$
Since Euler’s number,
$$e$$
, is a mathematical constant approximately equal to 2.718, another answer is:
$$12 \times 2.718=32.6$$
.
12
$$e$$
or 32.6
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Section: Logarithms
434. Logarithm
461. Log
465. Base Log
470. Natural Log
482. Ln Simplify
603. Bearing Type
604. Variable e
630. Type of Bearing