Material Yielding
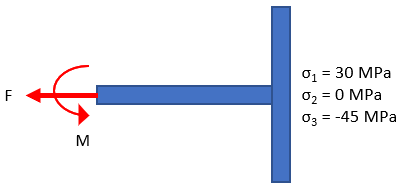
In the shown figure, a ductile beam is in tension due to an applied load, F, which creates the three corresponding principal stresses. Does failure occur if the material’s yielding strength is 80 MPa?
Expand Hint
Max shear stress:
$$$\tau_{max}=\frac{\sigma_1-\sigma_3}{2}$$$
where
$$\sigma$$
are the principal stresses.
Hint 2
Yielding occurs when
$$$\tau_{max}\geq \frac{S_y}{2}$$$
where
$$\tau_{max}$$
is the max shear stress, and
$$S_y$$
is the yield strength.
For a ductile material in tension, the max shear stress theory predicts that yielding will occur whenever
$$$\tau_{max}\geq \frac{S_y}{2}$$$
where
$$\tau_{max}$$
is the max shear stress, and
$$S_y$$
is the yield strength. To find the max shear stress:
$$$\tau_{max}=\frac{\sigma_1-\sigma_3}{2}$$$
where
$$\sigma$$
are the principal stresses. Thus,
$$$\tau_{max}=\frac{30MPa-(-45MPa)}{2}=\frac{75MPa}{2}=37.5\:MPa$$$
$$$\frac{S_y}{2}=\frac{80MPa}{2}=40\:MPa$$$
Because
$$37.5\:MPa\leq 40\:MPa$$
, failure does not occur.
Failure does not occur.
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Ductile Materials
274. Max Shear Stress Theory
Similar Problems from FE Section: Static Loading Failure Theories
274. Max Shear Stress Theory
640. Tensile and Compression Tests