Interval of Confidence
To test for yield strength, consider 100 steel test specimens were subjected to a tensile load test. If the standard deviation is 3 and the sample mean is 150 MPa, what is the 99% confidence interval for the steel’s yield strength?
Expand Hint
The population’s standard deviation,
$$\sigma$$
, is known, and the sample size is greater than 30. Thus, let’s use the z distribution. Only use the t distribution if
$$\sigma$$
is unknown and if the sample size is less than 30.
Hint 2
The Confidence Interval for the Mean µ of a Normal Distribution when a standard deviation
$$\sigma$$
is known:
$$$\bar{X}-Z_{a/2}\frac{\sigma}{\sqrt{n}}\leq \mu \leq \bar{X}+Z_{a/2}\frac{\sigma}{\sqrt{n}}$$$
where
$$\bar{X}$$
is the sample mean,
$$\sigma$$
is the standard deviation,
$$n$$
is the sample size, and
$$Z_a$$
corresponds to the appropriate probability under the normal probability curve for a given
$$Z_{var}$$
(standard normal Z score).
The population’s standard deviation,
$$\sigma$$
, is known, and the sample size is greater than 30. Thus, let’s use the z distribution. Only use the t distribution if
$$\sigma$$
is unknown and if the sample size is less than 30.
The Confidence Interval for the Mean µ of a Normal Distribution when a standard deviation
$$\sigma$$
is known:
$$$\bar{X}-Z_{a/2}\frac{\sigma}{\sqrt{n}}\leq \mu \leq \bar{X}+Z_{a/2}\frac{\sigma}{\sqrt{n}}$$$
where
$$\bar{X}$$
is the sample mean,
$$\sigma$$
is the standard deviation,
$$n$$
is the sample size, and
$$Z_a$$
corresponds to the appropriate probability under the normal probability curve for a given
$$Z_{var}$$
(standard normal Z score).
$$Z_{a/2}$$
is usually derived from confidence interval table (like in the FE exam handbook):
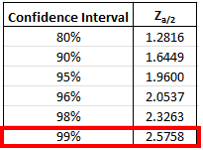
Therefore, the 99% confidence interval is:
$$$150-2.58\frac{3}{\sqrt{100}}\leq \mu \leq 150+2.58\frac{3}{\sqrt{100}}$$$
$$$150-2.58(0.3)\leq \mu \leq 150+2.58(0.3)$$$
$$$150-0.774\leq \mu \leq 150+0.774$$$
$$$149.226\leq \mu \leq 150.774$$$
Thus, the answer is (149.23, 150.77).
(149.23, 150.77)
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Confidence Interval for the Mean µ of a Normal Distribution
360. Confidence Interval
Similar Problems from FE Sub Section: Values of Z
360. Confidence Interval
Similar Problems from FE Section: Confidence Intervals, Sample Distributions and Sample Size
360. Confidence Interval
Similar Problems from FE Section: Test Statistics
360. Confidence Interval