Imaginary Roots
Find two roots that satisfy the shown equation:
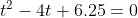
Expand Hint
Quadratic Equation Formula:
$$$x_1,x_2=\frac{-b\pm \sqrt{b^{2}-4\cdot a\cdot c}}{2a}$$$
Hint 2
Quadratic Equation Form:
$$$ax^2+bx+c=0$$$
Quadratic Equation Formula:
$$$x_1,x_2=\frac{-b\pm \sqrt{b^{2}-4\cdot a\cdot c}}{2a}$$$
Quadratic Equation Form:
$$$ax^2+bx+c=0$$$
The problem’s equation can be rewritten as:
$$$1x^2-4x+6.25=0$$$
Thus,
$$$x_1,x_2=\frac{-(-4)\pm \sqrt{(-4)^{2}-4\cdot (1)\cdot (6.25)}}{2(1)}$$$
$$$x_1,x_2=\frac{4\pm \sqrt{16-25}}{2}=\frac{4\pm\sqrt{-9}}{2}$$$
$$$x_1,x_2=\frac{4\pm3i}{2}=2\pm 1.5i$$$
2 ± 1.5i
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Section: Quadratic Equation
127. Quadratic Equation
383. Roots
560. Quad Equation