A Capacitor's Voltage

#069 / Electrical & Computer Engineering Medium

The shown diagram is a circuit connected to a 12V voltage source. What is the voltage across the capacitor? Assume steady state.

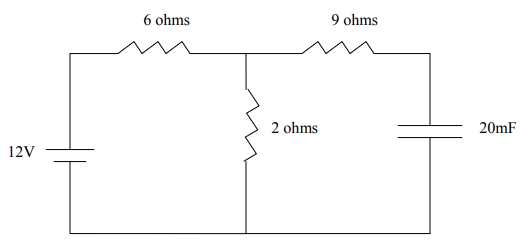
Expand Hint
At steady state, the circuit will act as if the capacitor is an open circuit. Since the capacitor is charged, no more current can flow through it. The 6Ω and 2Ω resistors are in the closed circuit.
Hint 2
Ohm's Law:
$$$V=iR$$$
where $$V$$ is the voltage, $$i$$ is the current, and $$R$$ is the resistance.
At steady state, the circuit will act as if the capacitor is an open circuit. Since the capacitor is charged, no more current can flow through it. The 6 Ω and 2 Ω resistors are in the closed circuit. For series connections, the current in all resistors is the same and the equivalent resistance for n resistors in series is:
$$$R_{series}=R_1+R_2+...+R_n$$$
Thus,
$$$R_{eq}=R_{1}+R_{2}=6+2=8\:\Omega $$$
Ohm's Law:
$$$V=iR$$$
where $$V$$ is the voltage, $$i$$ is the current, and $$R$$ is the resistance.

Using Ohm's law to solve for the circuit's current:
$$$i=\frac{V}{R_{eq}}=\frac{12V}{8\Omega}=1.5\:A$$$
Because no current is flowing through the 9Ω resistor, the voltage across the capacitor is the same across the 2Ω resistor.
$$$V=iR=1.5A\cdot 2\Omega=3\:V$$$
3 V


☕️ Donate to keep your study guide and instructor running!
🚨 [Beta] Test your FE exam readiness with a timed quiz!
☝️ Take the survey to help us improve!

< Prev #068

random #

#070 Next >