Thick Walled Vessel
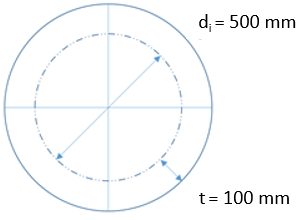
Consider an air cylinder’s pressure gauge reads 1,700 kPa. If the cylinder is made of a 100 mm steel rolled plate, with an internal diameter of 500 mm, what is the tangential (hoop) stress (kPa)? Assume a thick walled cylinder.
Expand Hint
For internal pressure only, the stresses at the inside wall are:
$$$\sigma_t=P_i\frac{r_o^{2}+r_i^{2}}{r_o^{2}-r_i^{2}}$$$
where
$$P_i$$
is the internal pressure,
$$r_o$$
is the outside radius, and
$$r_i$$
is the inside radius.
Hint 2
$$$r_o=\frac{500}{2}+100=350\:mm$$$
$$$r_i=\frac{500}{2}=250\:mm$$$
Because this is a thick walled vessel, the stresses caused by the internal pressure on the inside wall is:
$$$\sigma_t=P_i\frac{r_o^{2}+r_i^{2}}{r_o^{2}-r_i^{2}}$$$
where
$$P_i$$
is the internal pressure,
$$r_o$$
is the outside radius, and
$$r_i$$
is the inside radius. The radii are:
$$$r_o=\frac{500}{2}+100=350\:mm$$$
$$$r_i=\frac{500}{2}=250\:mm$$$
Solving for tangential stress:
$$$\sigma_t=1,700kPa\times \frac{350^{2}+250^{2}}{350^{2}-250^{2}}$$$
$$$=1,700kPa\times\frac{185,000}{60,000}$$$
$$$=5,242\:kPa$$$
5,242 kPa
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Thick-walled Cylinder
523. Thick Vessel
528. Internal Pressure
536. End Cap Vessel
Similar Problems from FE Section: Cylindrical Pressure Vessel
056. Tangential Stress
266. Thin Wall Cylinder
314. Hoop Stress
376. Pressure Cylinder
523. Thick Vessel
528. Internal Pressure
532. Axial Stress
536. End Cap Vessel