Tensional Force
Consider a construction crane is suspending a 50 kg payload in the shown diagram. What is the tensional force in each of the two cables holding up the load?
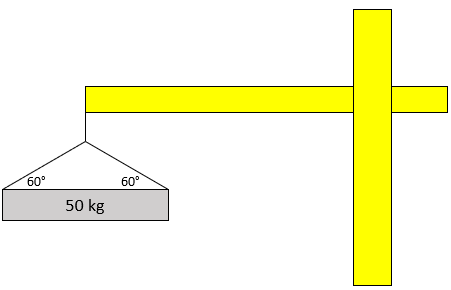
Expand Hint
The problem is asking to solve for the y-component of
$$F_{tension}$$
in the free body diagram:
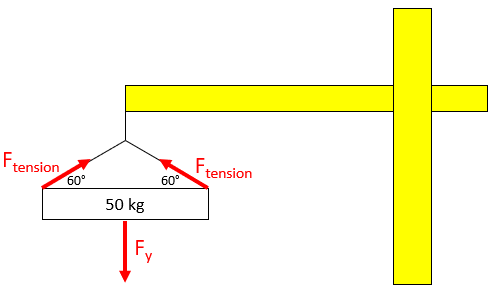
Hint 2
To find
$$F_y$$
, multiply the mass by gravity (Newton's 2nd law):
$$$F_y=mass\times acceleration$$$
The problem is asking to solve for the y-component of
$$F_{tension}$$
in the free body diagram:
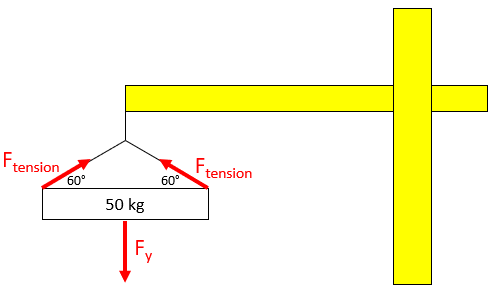
To find
$$F_y$$
, multiply the mass by gravity (Newton's 2nd law):
$$$F_y=mass\times acceleration=50kg\times 9.8m/s^2=490\:N$$$
Because there are two upward pulling cables in the vertical direction, they will counteract the entire downward force. Thus:
$$$F_y=(2)F_{tension}\cdot sin(60^{\circ})=490\:N$$$
$$$F_{tension}=\frac{490N}{2\cdot sin(60^{\circ})}=\frac{490N}{1.732}=283\:N$$$
283 N
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Force
055. Free Body Diagram
164. Pulleys
304. Vertical Force
310. Simply Supported
365. Beam AB
369. A Beam
380. Reaction Point
449. Tire Swing
656. Beam
Similar Problems from FE Section: Force
055. Free Body Diagram
145. Force Characteristics
164. Pulleys
304. Vertical Force
310. Simply Supported
365. Beam AB
369. A Beam
380. Reaction Point
449. Tire Swing
656. Beam
Similar Problems from FE Section: Systems of Forces
380. Reaction Point
449. Tire Swing