Law of Cosines
For the triangle figure shown, what is the length of the third side?
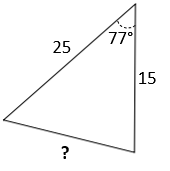
Expand Hint
Law of Cosines:
$$$b^2=a^2+c^2-2ac\:cos\:B$$$
Hint 2
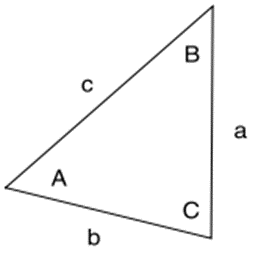
For oblique (non-right) triangles, use the Law of Cosines to determine unknown sides:
$$$a^2=b^2+c^2-2bc\:cos\:A$$$
$$$b^2=a^2+c^2-2ac\:cos\:B$$$
$$$c^2=a^2+b^2-2ab\:cos\:C$$$
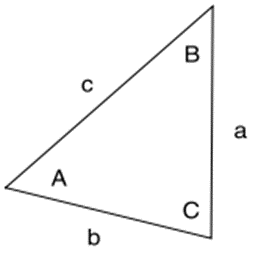
Thus, to find side
$$b$$
:
$$$b^2=a^2+c^2-2ac\:cos\:B$$$
$$$b^2=15^2+25^2-2(15)(25)\:cos\:77^{\circ}$$$
$$$b^2=225+625-(750)(0.2249)$$$
$$$b=\sqrt{850-168.71}=\sqrt{681.29}=26.1$$$
26.1
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Law of Cosines
390. Cosines
525. Cosine Law
529. Unknown Side
Similar Problems from FE Section: Trigonometry
390. Cosines
393. Law of Sines
525. Cosine Law
529. Unknown Side
533. Sines
537. Sin