Cosines
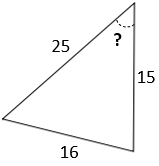
For the triangle figure shown, what is the unknown angle in degrees?
Expand Hint
Law of Cosines:
$$$b^2=a^2+c^2-2ac\:cos\:B$$$
Hint 2
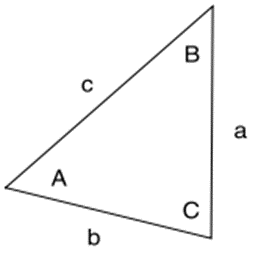
For oblique (non-right) triangles, use the Law of Cosines to determine unknown sides/angles:
$$$a^2=b^2+c^2-2bc\:cos\:A$$$
$$$b^2=a^2+c^2-2ac\:cos\:B$$$
$$$c^2=a^2+b^2-2ab\:cos\:C$$$
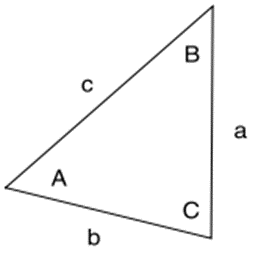
Thus, to find angle
$$B$$
:
$$$b^2=a^2+c^2-2ac\:cos\:B$$$
$$$2(15)(25)\:cos\:B=(15)^2+(25)^2-(16)^2$$$
$$$(750)\:cos\:B=225+625-256=594$$$
$$$cos\:B=\frac{594}{750}\Rightarrow B=cos^{-1}(\frac{594}{750})$$$
$$$B=cos^{-1}(0.792)=37.6^{\circ}$$$
37.6°
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Law of Cosines
346. Law of Cosines
525. Cosine Law
529. Unknown Side
Similar Problems from FE Section: Trigonometry
346. Law of Cosines
393. Law of Sines
525. Cosine Law
529. Unknown Side
533. Sines
537. Sin