Elongation

#174 / Mechanics of Materials Easy

A titanium rod with a cross sectional area of 20 cm^2 in the below figure is placed under tension. Determine the elongation if the Modulus of Elasticity (E) = 105 x 10^9 N/m^2.

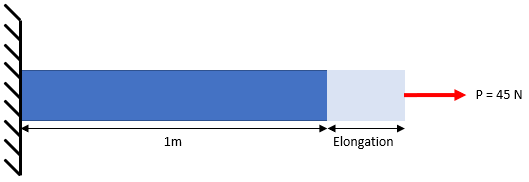
Expand Hint
$$$\delta =\frac{PL}{AE}$$$
where $$\delta$$ is the elastic longitudinal deformation, $$L$$ is the length of member, $$P$$ is the loading, $$A$$ is the cross sectional area, and $$E$$ is Modulus of Elasticity.
Hint 2
Remember to keep track of units when solving for $$\delta$$ .
Uniaxial Loading and Deformation:
$$$\delta =\frac{PL}{AE}$$$
where $$\delta$$ is the elastic longitudinal deformation, $$L$$ is the length of member, $$P$$ is the loading, $$A$$ is the cross sectional area, and $$E$$ is Modulus of Elasticity.

Plugging in the values (keep track of units):
$$$\delta =\frac{PL}{AE}=\frac{45N\cdot 1m}{0.002m^2\cdot 105\times 10^9\frac{N}{m^2}}=\frac{45m}{210,000,000}$$$
$$$\delta =2.14\times 10^{-7}\:m=0.000214\:mm$$$
0.000214 mm


☕️ Donate to keep your study guide and instructor running!
🚨 [Beta] Test your FE exam readiness with a timed quiz!
☝️ Take the survey to help us improve!

< Prev #173

random #

#175 Next >