Definite Integrals
Calculate the definite integral shown.
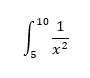
Expand Hint
$$$\int x^n \:dx=\frac{x^{n+1}}{n+1}$$$
Hint 2
After solving for the indefinite integral, plug in 10 as the upper integral boundary and 5 as the lower integral limit.
This is a two step calculus problem. First, let’s applying the power rule to solve the integral:
$$$\int \frac{1}{x^2}dx=\frac{x^{n+1}}{n+1}\:with\:n=-2$$$
$$$\int (x^{-2})dx=-\frac{1}{x}+C$$$
Next, solve using the upper integral boundary of 10, and lower integral limit of 5:
$$$(\frac{-1}{x})-(\frac{-1}{x})\rightarrow -\frac{1}{10}+\frac{1}{5}=-0.1+0.2=0.1$$$
0.1
Time Analysis
See how quickly you looked at the hint, solution, and answer. This is important for making sure you will finish the FE Exam in time.- Hint: Not clicked
- Solution: Not clicked
- Answer: Not clicked
Similar Problems from FE Sub Section: Integral Calculus
040. Integrals
043. A Calc Question
077. Area Under a Curve
308. Definite Integral
353. Inflections
408. Triple Integrals
416. Trip Integral
430. A Curve’s Area
643. Integral Finding
Similar Problems from FE Section: Differential Calculus
040. Integrals
043. A Calc Question
048. Derivative
051. 2nd Derivatives
077. Area Under a Curve
078. Derivatives
122. Roller Coasters
158. A Train's Velocity
308. Definite Integral
312. The Derivative
342. Inflection Point
353. Inflections
355. Multi Roots
394. Acceleration
408. Triple Integrals
412. Hill Bomb
416. Trip Integral
430. A Curve’s Area
643. Integral Finding
650. Differentiate