RFS Boundaries

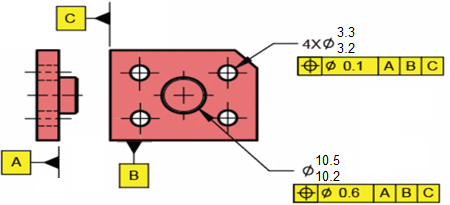
#421 / Mechanical Engineering Interview Hard

Based on the two position tolerances shown, what are the respective outer and inner boundaries for the 4x holes and 1x pin?

Expand Hint
The Inner Boundary (IB) is a worst case theoretical boundary created by subtracting the stated geometric tolerance (and any other applicable tolerances) from the smallest allowed feature (LMC for for external features and MMC for internal ones).
Hint 2
The Outer Boundary (OB) is a worst case theoretical boundary created by adding the stated geometric tolerance (and any other applicable tolerances) from the largest allowed feature (MMC for external features and LMC for internal ones).
The Inner Boundary (IB) is a worst case theoretical boundary created by subtracting the stated geometric tolerance (and any other applicable tolerances) from the smallest allowed feature (LMC for for external features and MMC for internal ones). The Outer Boundary (OB) is a worst case theoretical boundary created by adding the stated geometric tolerance (and any other applicable tolerances) from the largest allowed feature (MMC for external features and LMC for internal ones).
Because there are no feature modifiers (MMC, LMC etc.) specified in the feature control frame, the default, Regardless of Feature Size (RFS), applies to the problem, meaning additional bonus tolerances are not factored in when determining IB and OB. In summary,
For an Internal Feature (Hole) with Geo Tol at RFS:
  • IB = MMC (think smallest hole) - geo tol
  • OB = LMC (think largest hole) + geo tol
For an External Feature (Pin) with Geo Tol at RFS:
  • IB = LMC (think smallest pin) - geo tol
  • OB = MMC (think largest pin) + geo tol
where $$geo\:tol$$ is the stated value in the feature control frame.

Thus, the IB and OB for the hole are:
$$$IB_{hole}=3.2-0.1=3.1$$$
$$$OB_{hole}=3.3+0.1=3.4$$$
Finally, the IB and OB for the pin are:
$$$IB_{pin}=10.2-0.6=9.6$$$
$$$OB_{pin}=10.5+0.6=11.1$$$
$$$IB_{hole}=3.1$$$
$$$OB_{hole}=3.4$$$
$$$IB_{pin}=9.6$$$
$$$OB_{pin}=11.1$$$


☕️ Donate to keep your study guide and instructor running!
🚨 [Beta] Test your FE exam readiness with a timed quiz!
☝️ Take the survey to help us improve!

< Prev #420

random #

#422 Next >