Base Material

#636 / Materials Science Medium

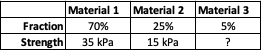
A composite material with a strain of 0.09 and a modulus of elasticity of 400 kPa is made up of three base materials from the shown table. What is base Material 3’s strength in kPa?

Expand Hint
Hooke’s Law:
$$$\sigma=E\varepsilon $$$
where $$\sigma$$ is the stress, $$E$$ is the elastic modulus (modulus of elasticity or Young’s modulus), and $$\varepsilon $$ is the strain.
Hint 2
$$$\sigma_c=\sum f_i\sigma_i$$$
where $$\sigma_c$$ is the strength parallel to the fiber direction, $$f_i$$ is the volume fraction of the individual material, and $$\sigma_i$$ is the individual material’s strength.
Hooke’s Law:
$$$\sigma=E\varepsilon $$$
where $$\sigma$$ is the stress, $$E$$ is the elastic modulus (modulus of elasticity or Young’s modulus), and $$\varepsilon $$ is the strain.
$$$\sigma =(400kPa)(0.09)=36\:kPa$$$
A composite material is a material formed from two or more “base” materials. The base/basic materials have notably dissimilar physical or chemical properties, but when merged to create a composite, produce properties unlike the individual elements. To find a composite’s strength:
$$$\sigma_c=\sum f_i\sigma_i$$$
where $$\sigma_c$$ is the strength parallel to the fiber direction, $$f_i$$ is the volume fraction of the individual material, and $$\sigma_i$$ is the individual material’s strength.
$$$\sigma_c=f_1 \sigma_1+f_2\sigma_2+f_3\sigma_3=36\:kPa$$$
Thus,
$$$36kPa=(0.7)(35kPa)+(0.25)(15kPa)+(0.05)\sigma_3$$$
$$$36kPa=24.5kPa+3.75kPa+(0.05)\sigma_3$$$
$$$\sigma_3=\frac{7.75kPa}{0.05}=155\:kPa$$$
155 kPa


☕️ Donate to keep your study guide and instructor running!
🚨 [Beta] Test your FE exam readiness with a timed quiz!
☝️ Take the survey to help us improve!

< Prev #635

random #

#637 Next >