Fracture

#538 / Materials Science Easy

A brittle specimen made out of Silicone Carbide has a stress intensity of 2,000 psi∙in^(1/2). What is the applied tensional force (psi) if the specimen developed the interior crack shown in the figure?

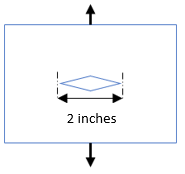
Expand Hint
Fracture toughness:
$$$K_{IC}=Y\cdot \sigma \cdot \sqrt{\pi (a)}$$$
where $$Y$$ is the geometrical factor, $$\sigma$$ is the applied engineering stress, and $$a$$ is the crack length.
Hint 2
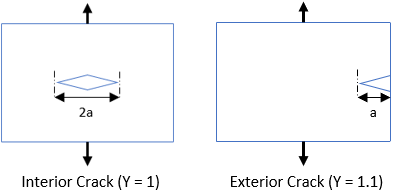
Fracture toughness is the stress intensity of when a brittle material will fail due to the combination of an applied stress and crack length.
$$$K_{IC}=Y\cdot \sigma \cdot \sqrt{\pi (a)}$$$
where $$K_{IC}$$ is fracture toughness, $$Y$$ is the geometrical factor, $$\sigma$$ is the applied engineering stress, and $$a$$ is the crack length.

Based on the problem statement:
  • $$K_{IC}=2,000\:\frac{lb}{in^2}\cdot in^{1/2}$$
  • $$Y=1$$ (since an interior crack was produced)
  • $$a=\frac{2in}{2}=1\:in$$
Solving for applied stress:
$$$\sigma=\frac{K_{IC}}{Y \sqrt{\pi (a)}}$$$
$$$\sigma=\frac{2,000\:lb\cdot\sqrt{in}}{in^2(1) \sqrt{\pi (1in)}}=\frac{2,000\:lb\cdot\sqrt{in}}{in^2 (1.772)\sqrt{in}}=1,128\:psi$$$
1,128 psi


☕️ Donate to keep your study guide and instructor running!
🚨 [Beta] Test your FE exam readiness with a timed quiz!
☝️ Take the survey to help us improve!

< Prev #537

random #

#539 Next >